Yesterday, I noticed that using the Pythia6 (embedding) response generated using pi0 triggers vs. using the Pythia6 response generated using charged hadron triggers to unfold our pi0 data makes a difference in the shape of the unfolded spectrum:

https://drupal.star.bnl.gov/STAR/blog/dmawxc/update-07222019-run-9-pp-comparing-effect-unfolding-data-using-embedding-response-differ

In particular, the shape of the unfolded spectrum (using the pi0-triggered Pythia6 response) compares favorably with the shape of the unfolded spectrum using the Pythia8 response (which is generated using pi0 triggers).  So a natural question is "how do the responses compare between the pi0-triggered Pythia6 case and the pi0-triggered Pythia8 case?"

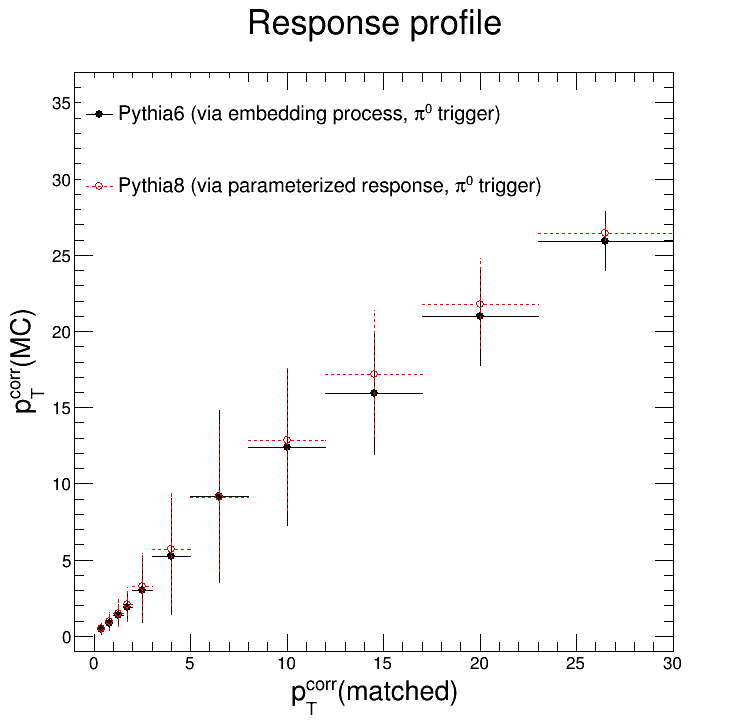
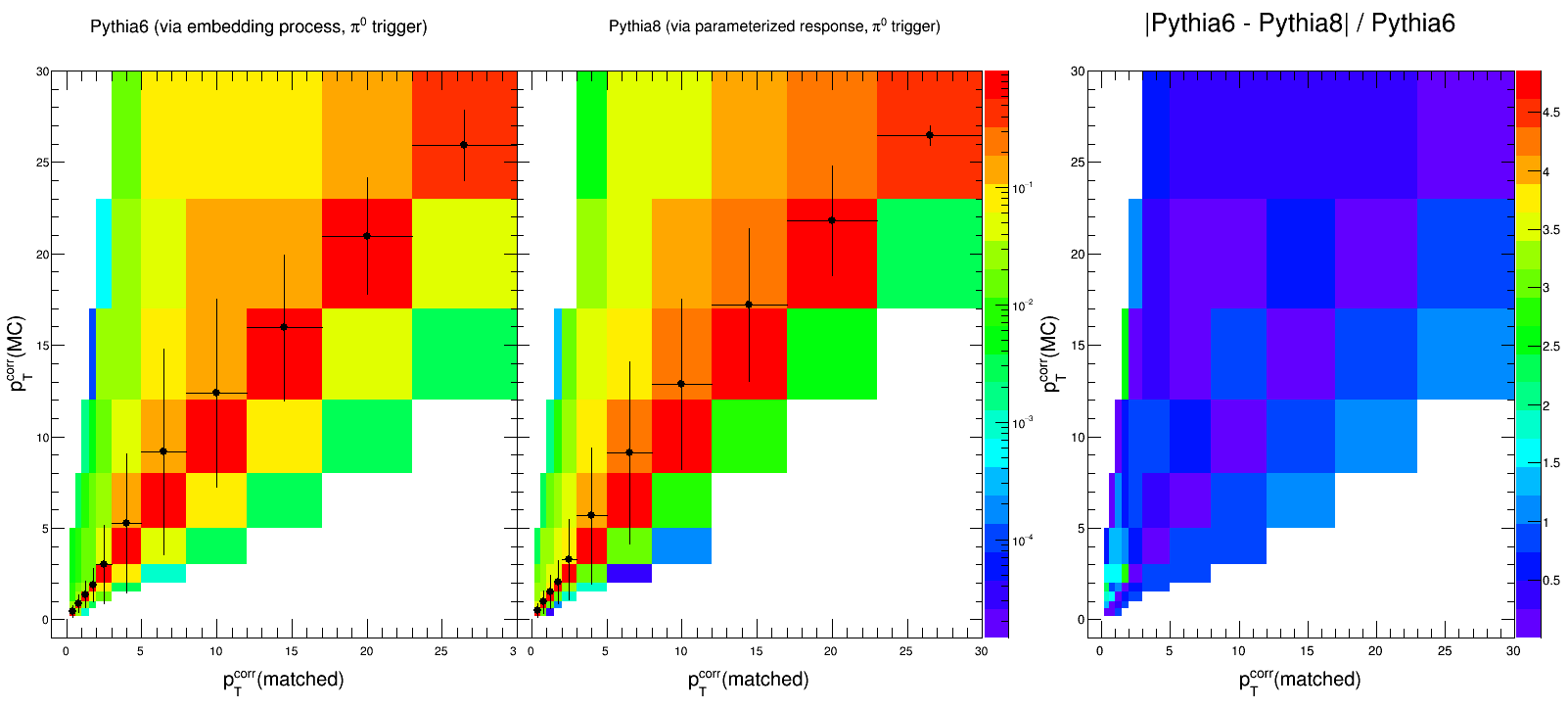
The efficiencies are definitely consistent, but -- intriguingly -- the response profile of the Pythia8 response matrix is harder than the Pythia6 response matrix (that is to say, the barycenters of the Pythia8 profiles lie higher on the particle-level pTjet axis than do the barycenters of the Pythia6 profiles).  And the fact that the unfolded data is harder if I use the Pythia8 response than the Pythia6 response is exactly what I'm trying understand right now.

I've seen this behavior before; for instance:

https://drupal.star.bnl.gov/STAR/blog/dmawxc/update-06102019-run-9-embedding-pythia8-vs-pythia6-responses-after-all-fixes

So why is this the case?  Well, since the efficiencies agree, that's probably not the issue.  Could it be the track resolution?  This is something we haven't checked; we always assumed that the resolution is a negligible effect.  But we do know that a finite resolution will make a spectrum appear harder, so that might very well be the effect here...  I will follow up soon...