Update 08.20.2018 -- Run 9 pp: a few checks on the gamma-rich purity
Following up on the previous study of the Run9 gamma-rich purity:
https://drupal.star.bnl.gov/STAR/blog/dmawxc/update-08052018-run-9-pp-purity-function-ettrg-and-zttrk
https://drupal.star.bnl.gov/STAR/blog/dmawxc/update-08152018-run-9-pp-purity-function-zttrk-bins-ettrg
A natural question is what happens when the cuts on pTtrk are opened up. In jet reconstruction, we use pTtrk = (0.2, 20) GeV/c, but in the above calculation we used pTtrk = (1.2, 20) GeV/c. And so below is the purity for pTtrk = (0.2, 20) GeV/c.
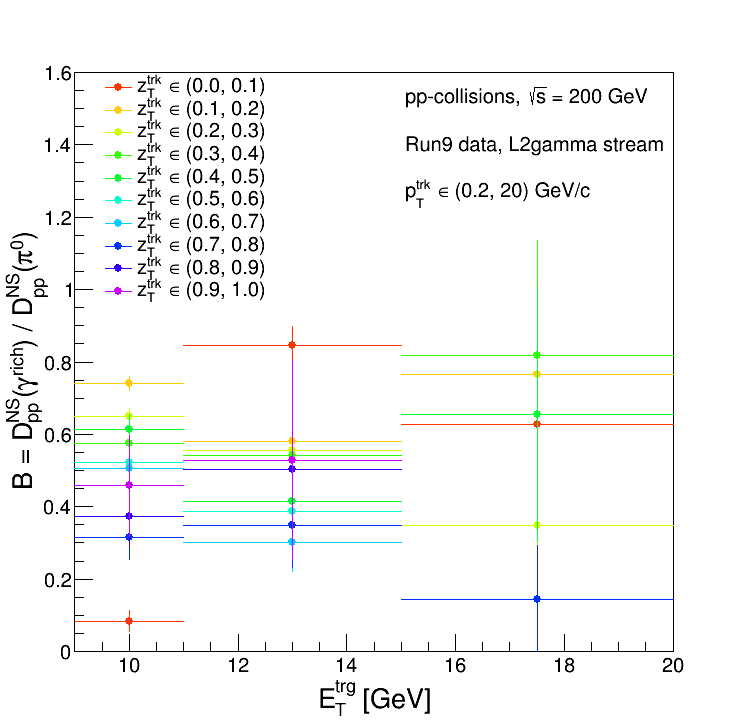
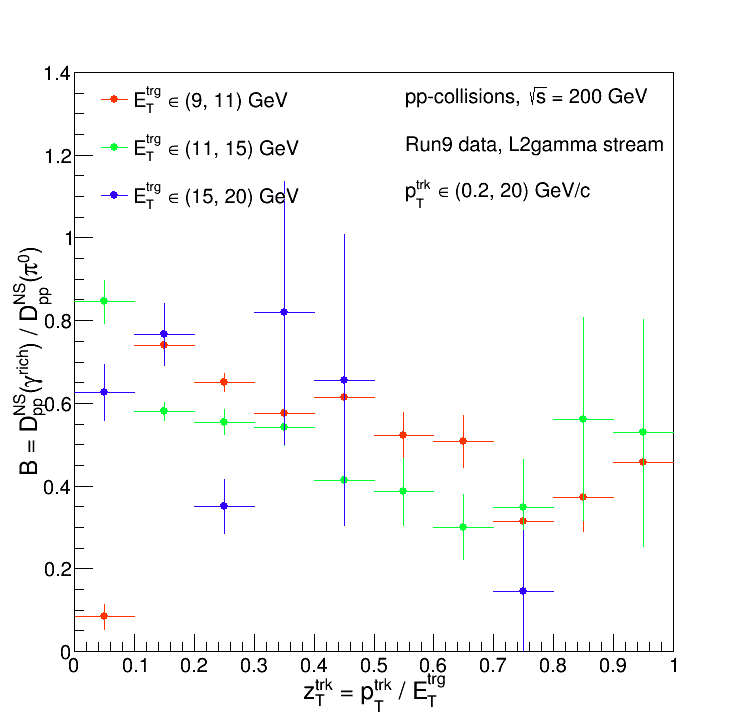
For the previous we calculations we typically used zTtrk = (0.2, 0.3), so here are the values of B for zTtrk = (0.2, 0.3) for both pTtrk ranges:
| pTtrk = (0.2, 20) GeV/c | pTtrk = (1.2, 20) GeV/c | |||
| eTtrg | B | error | B | error |
| (9, 11) GeV | 0.650 | 0.022 | 0.650 | 0.022 |
| (11, 15) GeV | 0.554 | 0.030 | 0.554 | 0.030 |
| (15, 20) GeV | 0.350 | 0.065 | 0.350 | 0.065 |
So, as expected, they're the same: requiring zTtrk = (0.2, 0.3) means that only tracks with pTtrk = (1.8, 6) GeV/c are used. Lastly, I calculated the purity vs. eTtrg for zTtrk = (0.2, 0.3) with pTtrk = (1.2, 20) GeV/c:
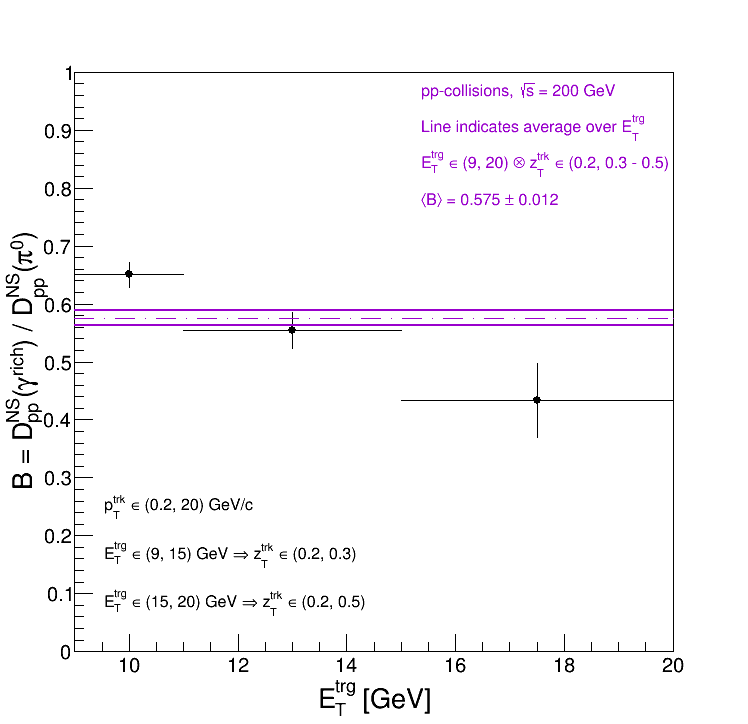
Note: I've also attached equivalent plots requiring pTtrk = (0.2, eTtrg) GeV/c. Since we also require zTtrk cuts (up to zTtrk = 1), they're identical as expected.
Update [08.20.2018]: the labels on the last plot above, and (previously) the text stated that zTtrk = (0.2, 0.5) was used for eTtrg = (15, 20) GeV with zTtrk = (0.2, 0.3) for the other 2 bins. Accidentally, I applied zTtrk = (0.2, 0.3) for all 3 bins. To see the eTtrg-dependent zTtrk cuts, see this post:
https://drupal.star.bnl.gov/STAR/blog/dmawxc/update-08202018-run-9-pp-purity-testing-zttrk-cuts
- dmawxc's blog
- Login or register to post comments
