Toward W Cross Section for APS

I took the quantities from Ross's writeup to get some very preliminary efficiencies from our current (private) MC.  There are several things incorrect about this sample: no 'real' status tables for TPC and B/EEMC, narrow vertex distribution, old geometries, etc.  But it gives us a crude look at what our efficiencies are, and sets up the software to calculate them correctly when the new MC samples arrive.

Note: The errors are given by err=sqrt(A*(B-A)/(B)^3), where B is the number of possible events and A is the number of accepted events.

Fig 1) Trigger Efficiency:  Likelihood of event with electron |eta|< 1 to pass trigger

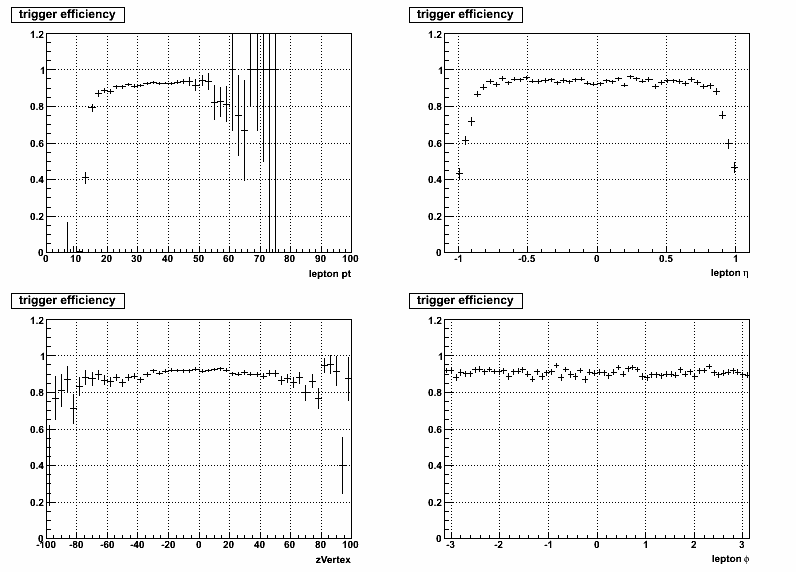
Fig 2) Vertex Efficiency:  Likelihood of event with good trigger to have a good reconstructed vertex (rank > 0 && |zVertex| < 100)

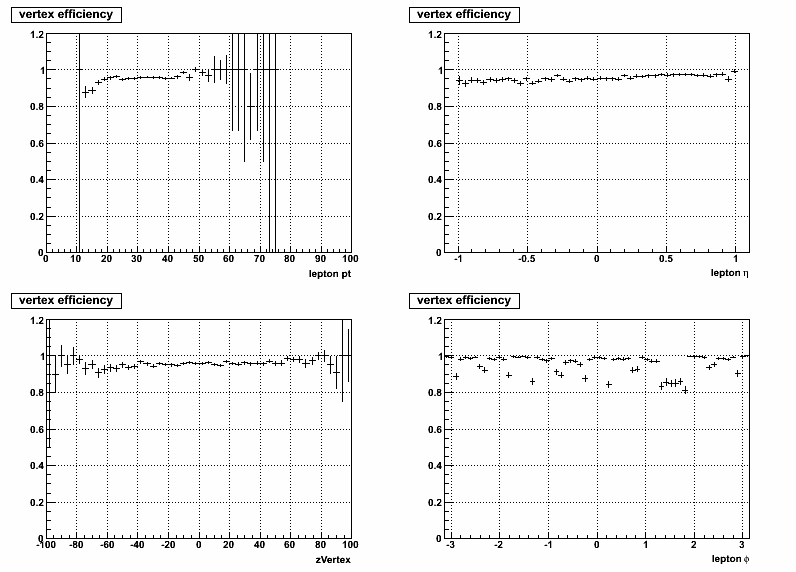
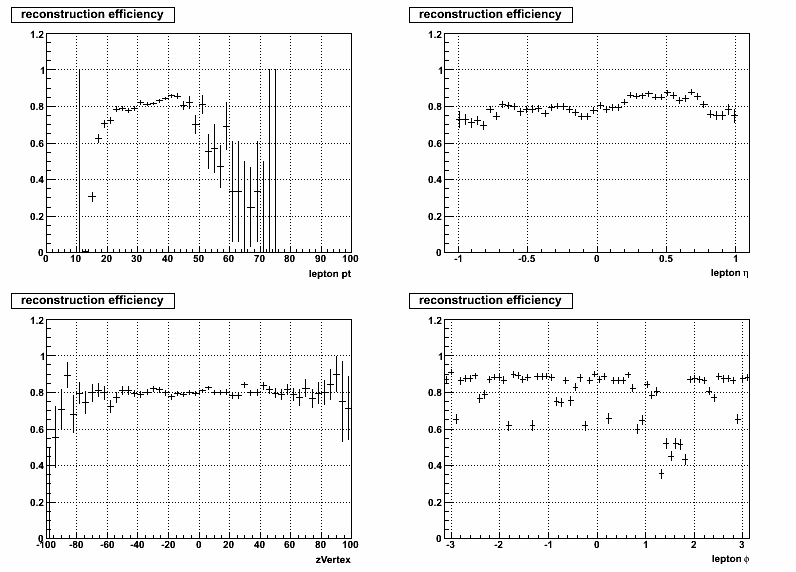
Fig 3) Reconstruction Efficiency:  Likelihood of event with good vertex to pass all the cuts in W algo

Below is a very preliminary estimate of the charge summed W cross section.  Here are the assumptions:

    1) Luminosity from setABCD = ~12 pb^-1

    2) Efficiency:  constant ~68% (from above: trig * vert * reco = 0.9 * 0.95 * .8)

    3) Background subtracted yield similar to Jan's presentation at Austin (with additional requirement that the electron track |eta| < 1)

Fig 4) Background subtracted yields (with requirement electron track |eta| < 1)

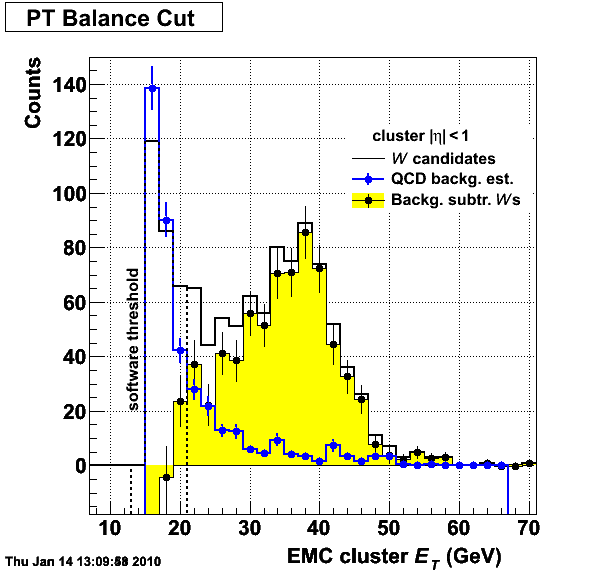
Fig 5)  Charge Summed W cross section (only statistical errors are shown)

Cross Section binned in |eta|

Fig 6)  Background subtracted yields in 3 |eta| bins

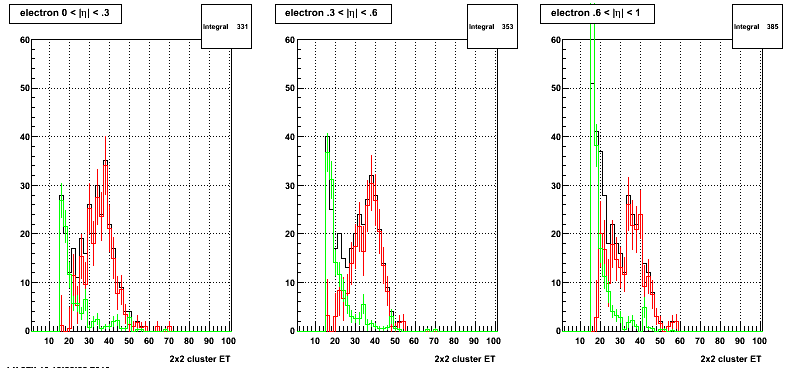
For the two smaller |eta| bins it looks like our background subtraction is working very well.  However, for the largest |eta| bin the shape of the ET distribution is different.  We expect the distribution to change as we move to larger |eta|, right?  How does this affect our background subtraction technique.

Assumed luminosity ~ 12 pb^-1 and overall efficiencies (from fig 1, 2, 3 above)

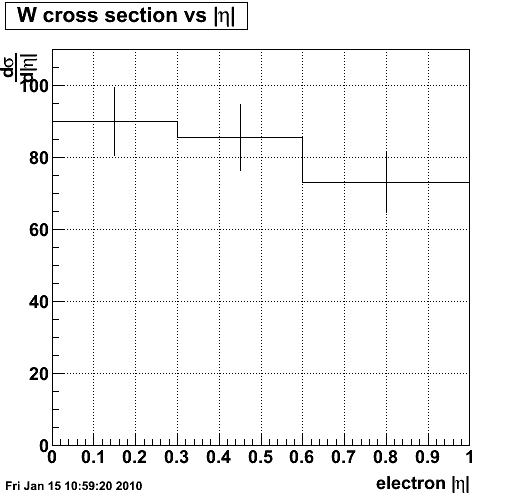
Fig 7)  W cross section vs |eta| (only statistical errors are shown)