There has been extensive discussion of how to optimize the 200 GeV running.
I did a few caculations quantifying this. Some basic

• assume initial vertex distribution is Gaussian with sigma of 50 cm
• assume beam width at z-0 is 150 microns
• The hourglass effect implies that sigma(z)=sigma(0)*sqrt(1+z*z/beta*beta)) where beta is the beta*, nominaly 0.7 m at 200 GeV.
• Assume that the beam have a crossing angle of 0.0, 0.001,0.002 mrad
• Reduction at given Z is reduced by Gaussian dist of width(z) vs. seperation

The results can be seen in the following Graph

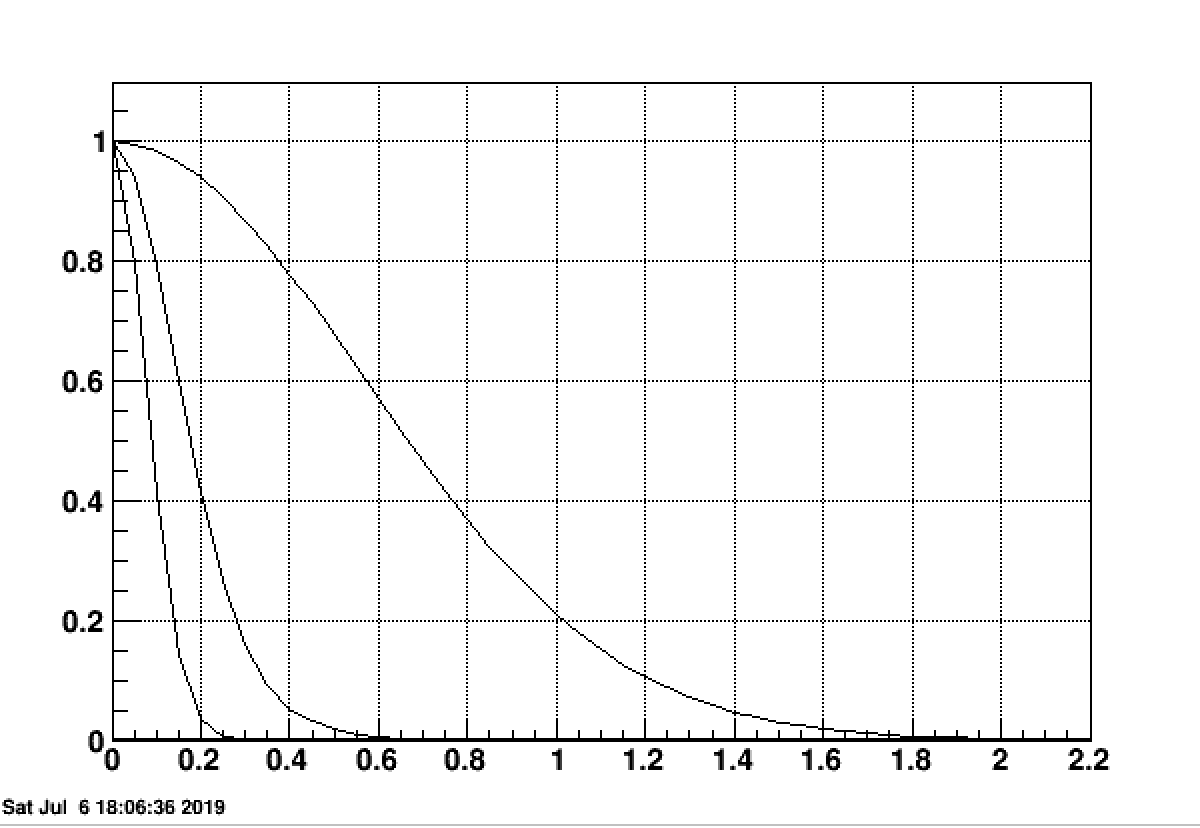
A couple of comments to think about

• The vertex distribution are much narrower with the crossing angle is already pointed out. The  luminosity  for the reduced widths are 30% for 0001 and 16% at 0.002 of the nominal lumi.
• The intrinsic divergence in the beam has not been considered
• With a crossing angle collisions there is center-of-mass transverse velocity. This will e.g for crossing angle of .002 change the measured pt of pions at 0.5 GeV/c by 1 MeV/c in the plane (+- in opposite hemispheres). This should be acceptable.
• The estimates do not take into account the intrinsic divergence of the beam, so the effective widht may be underestimated.