Mike proposed using a linear weighting method for the 16 EPD rings (combining E+W) at: drupal.star.bnl.gov/STAR/blog/lisa/ring-weights-estimating-global-quantities-linear-sums

Unfortunately the resolution was not as good as we expected.  His suggestion was to add a bias term, which gives the formalism below:

Our observable X is simply a weighted sum of the ring contents plus a bias term:

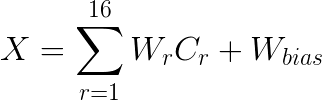
Then we will minimize the chi^2 as defined as the difference between our observable and some global variable, G_i:

The derivative of this with respect to W_r,W_bias will be zero to maximize the choice with respect to the global variable.

and

Which gives us an equation that looks identical to Mike's:

Where W_{17} = W_{bias}, C_{16,i} = 1 and

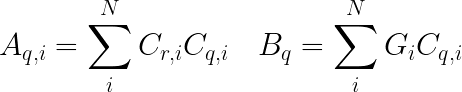
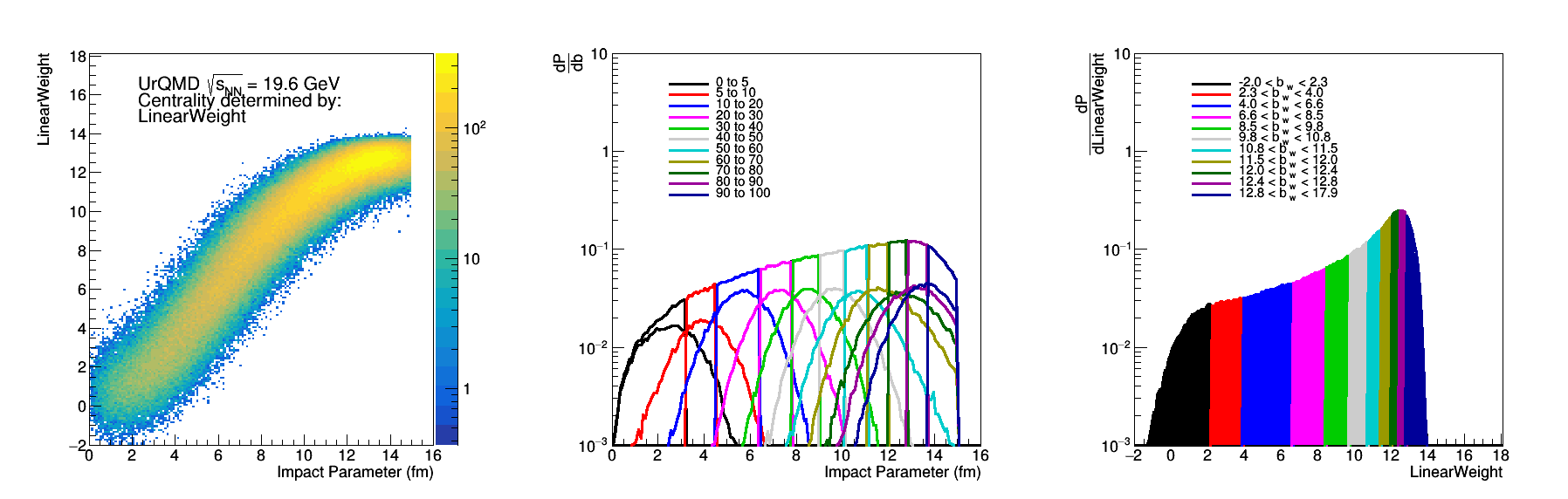
Figure 1: Linear Weight

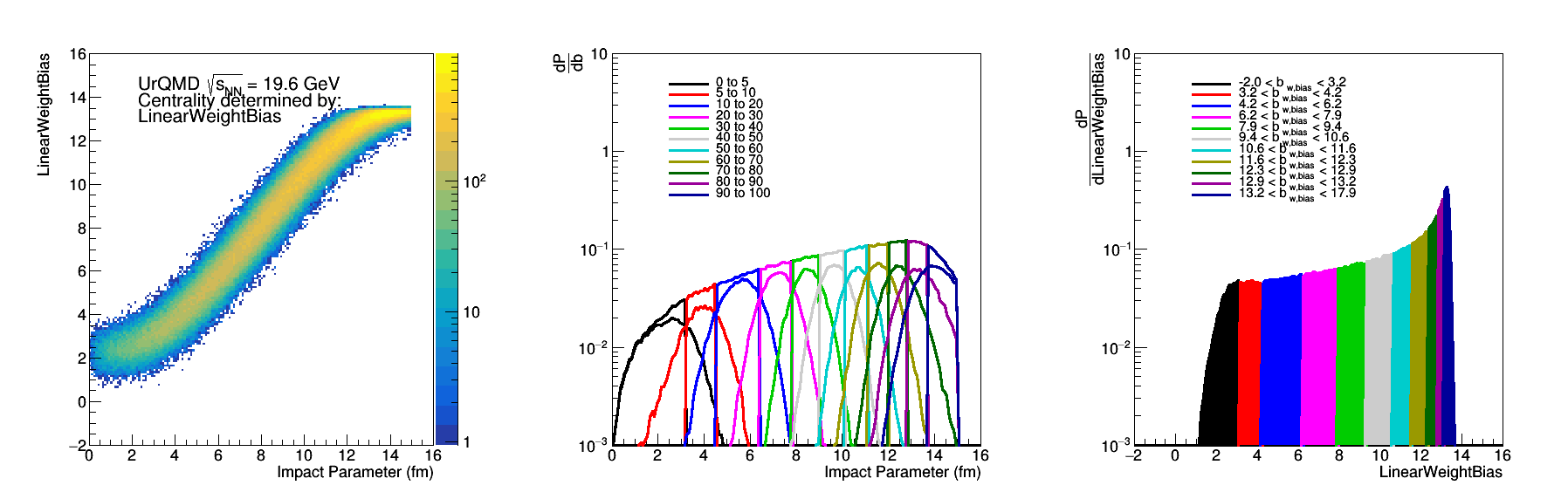
Figure 2: Linear weight + bias term

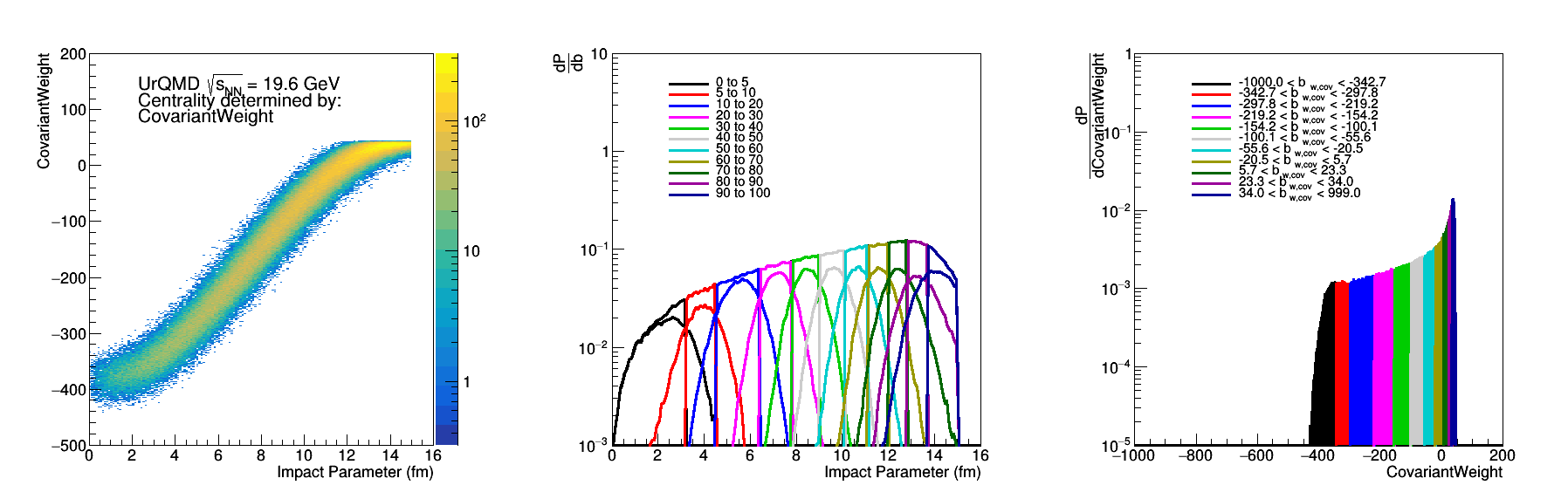
Figure 3: Covariance Weight

Figure 4: Linear Weight Particle

Figure 5: Linear Weight EPD (Nmip sum)

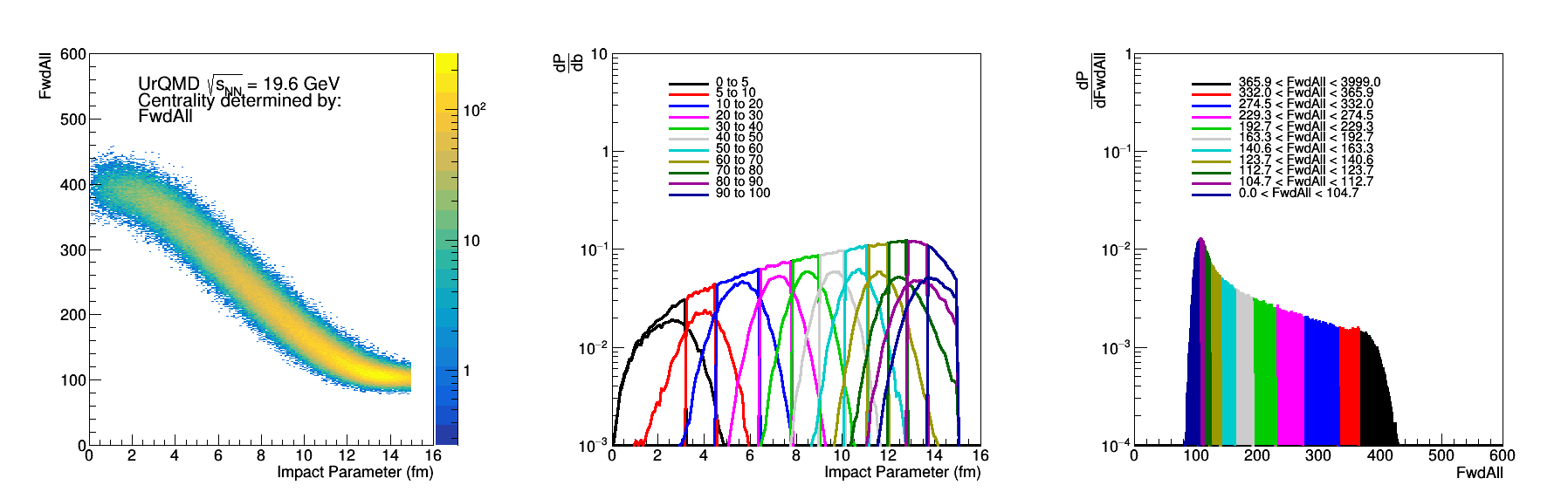
Figure 6: Fwd All

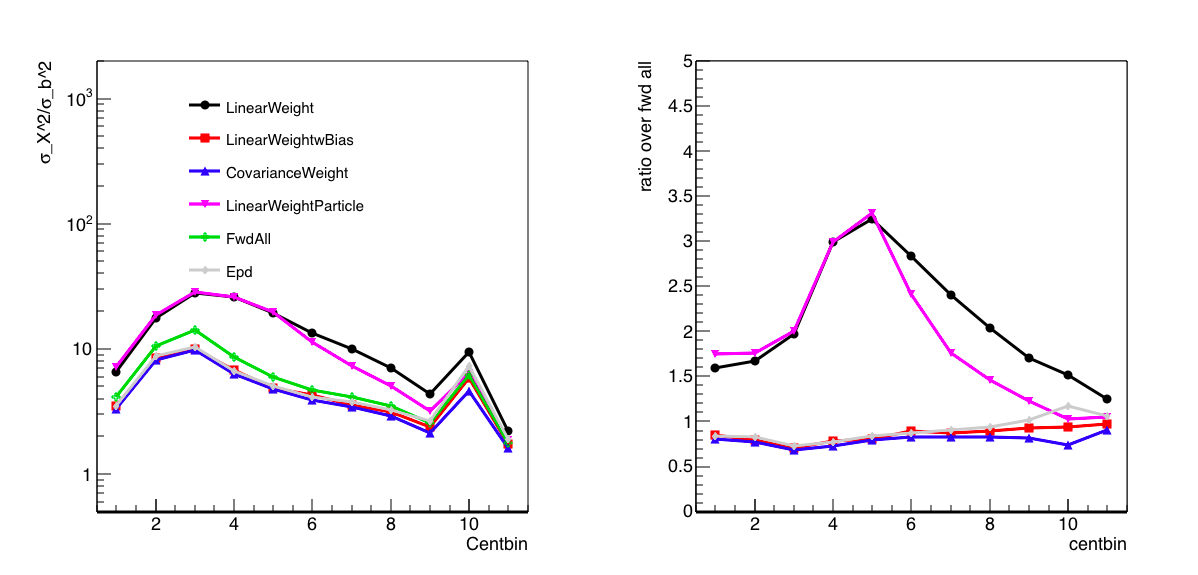
Figure 7: Left is the ratio of sigma_x^2/sigma_b^2.  The right is the ratio of the figures on the left over forward all.